
In order to consider the boundary of the physical, I first need to say what I mean by the physical. This explanation will in turn require visiting the topic of what the philosopher Wilfred Sellars called the “scientific and manifest images of the world”, and then, briefly, the question: “what is mathematics?”
The Scientific Image
The world as known to science can be seen as a kernel of fundamental physics, wrapped like the layers of an onion by mathematical structures, each with its own vocabulary and laws. For humans, prominent layers are 1) fundamental physics 2) chemistry 3) biology 4) neurology 4) the mental. For computers, corresponding layers are 1) fundamental physics 2) circuitry 3) software 4) application. I am setting aside other wrappings, such as the mechanics of macroscopic bodies and cosmology, for the purposes of this note.
Why do I designate these as mathematical layers? The key phrase is “the world as known to science”. Modern science has only to do with structure, not substance — it can only say what regularities are present, not what they are regularities of. Since the topic is always structure, and mathematics is the discipline that studies all possible structures, science is primarily a matter of finding mathematical structure in what we observe.
The above is a variant of what Wilfred Sellars calls “the scientific image” of existence, where quantum fields are fundamental, and the other layers are derived. In constrast, according to the older “manifest image”, the things that we directly experience, such as chairs and rocks, are basic. Bringing these two images together into one consistent view has proved difficult or impossible. It is one of the many formulations of the hard problem of consciousness: how does experience arise in the world considered scientifically?
What is Mathematics?
Let me quickly explain the various attitudes that have been held about the status of mathematics. First let’s make a distinction between mathematical language, and mathematical structures (also called mathematical objects). Mathematical language consists of strings of symbols that make statements about mathematical objects. The objects are things like numbers, geometric shapes, and fancier things like groups and manifolds. Now, mathematical platonists, also called mathematical realists, grant mathematical structures a reality independent of the human mind. Nominalists deny the existence of mathematical objects, and view mathematics as only a matter of language — of symbol manipulation. Intuitionists regard mathematical objects as mental constructions; no mathematical structure exists until it has been constructed by a human mind.
Mathematics and the Onion
The onion model requires at least partial platonism, it seems to me. The layers cannot just be mental constructions or imaginary constructs associated with a game of symbols, if they are to constitute aspects of the world which nearly all scientists would call real and external to humans. This does not commit us to full platonism (we might still be suspicious of the status of large cardinals, for example).
I am not saying, as Max Tegmark does, that the physical world is constituted by mathematics, but I am saying that the scientific image of it, built over centuries, asserts that certain mathematical structures are present within the physical world that we observe. Of course,the mathematical structures and layers as defined by science change from time to time. But at each point in time it is posited that some such structures exist, and this idea is what requires a degree of platonism. And indeed, at each stage, observations of the world at that stage have shown consistency with scientific models at the stage, so the world at each stage does indeed have structures within it that produce such consistency. For example, astronomical observations, suitably restricted, still cohere with Newtonian physics. In this sense the structures of Newtonian physics persist in the world, even if they are now seen as less fundamental.
From the onion viewpoint, then, a question is: what is special about the human and computational layers that gives them the status of “physically real”, if they are just one set of mathmatical structures among many, most of which we would not think of as physical (like the class of all sets or the monster group)? A possible answer: the layers have two special properties that set them apart: 1) the vocabulary and laws of each layer emerge from the layer below, at least in theory, and 2) each is temporal, in that time passes at the layer, and is internally causal in that there are regularaties, explanations, and predictive laws that apply within the layer. In other words, each of the layers has internal causal properties in the broad sense that things can be known about the layer’s future from knowledge about its past (both expressed in the vocabulary of the the layer). Note that time needs a relativistic definition at the fundamental layer but relativistic considerations are irrelevant for most situations encountered in other layers.
The first property grounds the layers, recursively, in the kernel of fundamental physics, and the second is what grants them the status of mathematical systems of scientific interest. Now, I have finally gotten to the point of defining the physical: it consists of the causal realms rooted in physics.
In Sellar’s manifest image, the fundamental elements of the physical are different :they are chairs, tables, persons, rocks, and so forth. So, we inhabit a world several layers of the onion out from what is fundamental from the standpoint of the scientific image. Presumably though, the manifest image nests within the structures that I have called physical, rather than floating in some other mathematical space. But I will leave the manifest image and its mysteries behind for the duration of this note, and concentrate on the scientific one.
The Boundary
By a boundary structure in this context I mean a structure within the physical, which exhibits a causal influence from outside the physical. My concern in this note is to undertake, via two examples, a brief exploration of this boundary.
A first and obvious point on the boundary is the mind of the mathematician, which contemplates structures on the far side of the physical/mathematical boundary, but is (presumably) implemented via a brain in the physical world. There is a sense in which the nature of the mathematical structures under contemplation, which are often outside the physical, cause the thoughts and writings of the mathematician about them, thereby forming a bridge between the physical and otherwise non-physical realms of the mathematical world. This view of things depends on the platonic attitude towards the mathematical structures involved. To take a simple case, consider the statement “Joan (or her phone) says that 53 times 14 is 742 because 53 times 14 is 742, and because Joan knows arithmetic (resp. the phone is correctly programmed for arithmetic)”. This is a correct statement, from the platonist’s point of view: The numbers 14, 53, and 742, possess their nature and properties in the human-indepenent world of mathematical objects. Therefore the statement “53 times 14 is 742” can serve as a fact in a chain of causation in this example, without circularity. It would also be correct to say, speaking in the vocabulary of another layer, “Joan (or her phone) says that 53 times 14 is 742 because this particular series of neural events occured in Joan’s brain (resp. electronic events in the phone’s circuitry).” There is no contradiction in having multiple correct causal explanations at different layers.
But there is a second, less obvious, point of contact. Consider the following image:
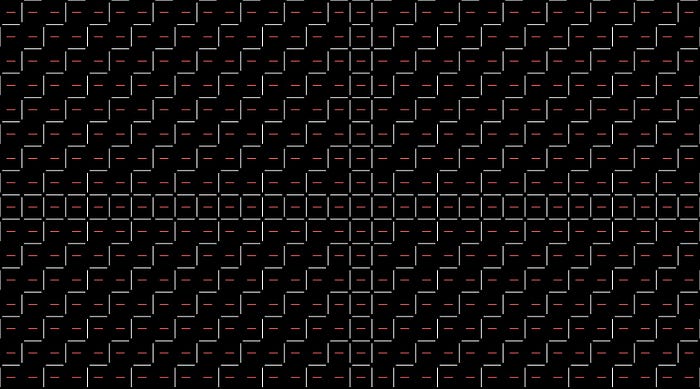
This image exhibits a pattern of an abstract kind. With a glance, you know all about it. If I asked you to describe it, you would likely come up with something like: “It is a grid with short red horizontal lines at the centers of cells. White lines consisting of the left and top boundaries of grid cells zigzag up and to the right across the grid, but there is also a horizontal band half way down where all four boundaries appear as lines, and a vertical band half way across with the same property. ” All of these aspects are available in your experience of the image within a second or so of seeing it. This is very close to an exact mathematical description of the image, in that it could easily be rendered in mathematical terms by someone with knowledge of analytic geometry and in fully formal terms by someone who is also familiar with axiomatic (eg ZF) set theory. That is, the experience that the image induces in your mind exhibits mathematical structure, whether or not you know how to express it in formal terms, and that structure is built immediately upon attending to the image. If this were not the case, no such description would be possible.
Now, my claim is that the pattern itself, as it exists externally to you, should not be classed as something physical, but only as having the kind of mathematical existence granted by the platonist to any mathematical structure. Thus, the recognition of the pattern by an observer constitutes another structure on the boundary of the physical.
The reason is this: the fact that a particular mathematical pattern is exhibited by the pixels of the above image has no causal effect, except when that pattern is recognized by an observer (human or computational). The pixels have their usual sort of causal effect, causing light to scatter in a given way, but without an observer there is no point at which the pattern plays a role. That is, if the set of mathematical patterns exhibited by pixels is regarded as a layer above the physical, then this layer of patterns, although emergent, has no internal causation associated with it, and therefore fails the test for the physical given earlier in this note. Thus, the observer of a pattern serves as a bridge between the physical and non-physical, just as does the mathematician. Specifically, the pattern causes mental and linguistic events in the observer, and thus the observer plays the role of a bridge from the non-physical.
Patterns of sound as well as sight, such as the patterns found in music, are non-physical as well.
